Berlin, DEU
Transport Network Efficiency (β)
a generalizable and scalable index for assessing routing efficiency on networks
Over 80%
of world's GDP generated in cities1
Over 4 billion
people live in urban areas2
nearly 90 billion USD
lost in traffic annually in the US alone3
How to measure a city's transport/routing efficiency?
Transport Network Efficiency
Addis Ababa, ETH
Bogota, Colombia
How does it work?
Consider a Traveling Salesperson Problem (TSP) traversing a Set of N i.i.d. demand points with X generated from a distribution f(x), where the support of f(x) is R, the well-known Beardwood-Halton-Hammersley (BHH) Theorem4 provides an estimate for the optimal TSP tour length:
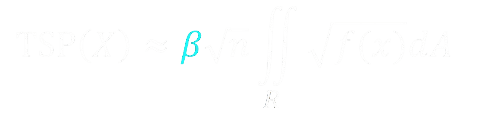
4Beardwood, J., Halton, J.H., Hammersley, J.M.: The shortest path through many points. Mathematical Proceedings of the Cambridge Philosophical Society 55(4), 299–327 (1959).
When applying BHH on a network,
TSP constant β provides a concise measure of the network’s routing efficiency
How it works
- Generate graph representation of urban networks
- Generate TSP samples from empirical demand distribution
- β estimation via BHH formulation
G(V,E)
Inferring data from β
- Dataset of worldwide transportation network efficiency index
- Engineer a feature set to explain network efficiency (i.e. β)
- Explaining β using network features
β
Short Distance Commute Efficiency
Long Distance Commute Efficiency
Distribution Uniformity
Node Density
Probability of Dense Subgroups